- Bridging the Terahertz Gap
- Modeling the Lithium-Ion Battery
- Protection contre la Corrosion
- Modélisation des batteries
- Modélisation et Simulation dans le développement des piles à combustible
- Modélisation thermique des petits satellites
- Analyse électro-vibroacoustique d'un transducteur à armature équilibrée
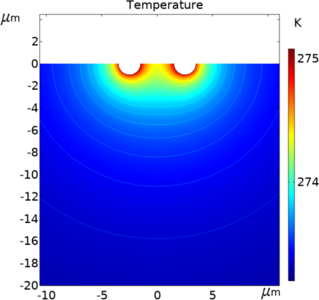
Time-dependent temperature response in bulk materials from two heat sources
Four-point probe resistance measurements on the microscale can induce significant localized heating which can be a source of measurement error, or an opportunity to measure thermal properties of materials. The amplitude and phase delay of the temperature affect micro four-point probe measurements performed using lock-in technique. Thus, it is important to model the time-dependent temperature distribution as accurately as possible. An analytical solution was developed and verified numerically, using finite element modelling in COMSOL Multiphysics®. We described the temperature distribution analytically in a bulk material heated by a harmonic external heat current source by solving the heat equation for a semi-infinite substrate. The solution predicts a temporal temperature distribution that follows a harmonic signal with a phase delay with respect to the external heat source signal. The phase delay is determined by the driving frequency of the external heat source, the distance from the source, and the thermal diffusivity of the material. The problem is then expanded to include two heat sources corresponding to contact probes that could be used to inject an electrical current into the substrate. The injected current results in Joule heating at the contact interface between the probes and bulk material. The analytical solutions are verified against numerical calculations. The COMSOL Multiphysics® Heat Transfer Module is used to solve the heat equation with matching boundary conditions to the analytical problems yet in a sample with finite geometry. A good agreement between the analytical solutions and the numerical calculations made with COMSOL Multiphysics® is found.