Articles techniques et présentations

Flash floods are sudden and mostly destructive rushes of water down narrow gullies or over sloping surfaces. They are mostly caused by heavy rainfall in the upstream watershed. They may also appear as result of catastrophic events as dam or levee breaks, or mudslides or debris flow.

The occurrence of flash floods is determined by many factors, like intensity, location and distribution of the rainfall, topography, land use and vegetation cover, soil type, and soil saturation. These factors determine the spatial and temporal development of a flood. Urban areas are also prone to flash floods, because impervious surfaces prevent water to infiltrate into the ground. Moreover damages are more severe in highly populated areas than in the remote countryside.

The simulation of flash floods has thus become a tool that is increasingly used. One application area is urban planning. In general flood modeling is utilized to delineate flood risk maps. Moreover such tools are applied in early warning systems, in order to predict the rush of a fluid front as reaction of a certain rainfall event.

Most modelers prefer the 2D depth averaged formulation in flash flood simulations in contrast to the general 3D formulation. The 2D version definitely has advantages concerning the general formulation with respect to computer resources. Near the fluid front mesh refinement is required, which highly increases the computational cost of 3D models.

The 2D approach leads to the shallow water equations (SWE), also known as Saint-Venant equations. Vertical velocities are usually much smaller than horizontal components, and are thus neglected. SWE consists of three coupled differential equations, one for the height of the water column and two for the mean horizontal velocity components. The system can be easily implemented in COMSOL Multiphysics® using the PDE interfaces.

In our contribution we present a systematic exploration of COMSOL Multiphysics® solutions of the SWE using dam break benchmarks. For the 1D classical benchmark we check numerically computed shock wave heights and velocities by the analytical solution. It turns out that numerical solutions become inaccurate for small backwater depths. Shock wave heights are not well predicted if the ratio backwater height/reservoir height becomes lower than 0.01. These errors are independent of the numerical approach, i.e. element type or discretization method.

However, for the usual parameter range, both in 1D and 2D, adaptive meshing techniques lead to accurate solutions requiring much less computational resources than simulations on fixed meshes. In 2D adaptive meshing reduces the model size by almost one order of magnitude, and the execution time by a factor of 20.

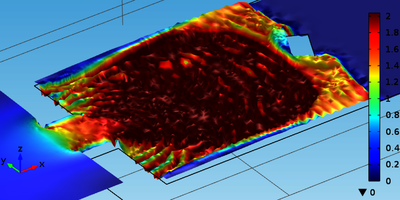
The figure depicts the flood at a time instant after a dam break within a channel containing an obstacle. The 3D figure shows the water depth distribution, computed for the 2D benchmark.