Articles techniques et présentations

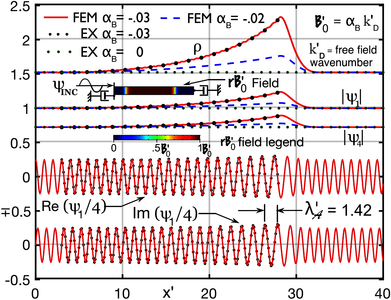
INTRODUCTION: The Dirac equation is employed in particle physics and historically gave the first combined unification of quantum mechanics and relativity theory by introducing a four component wave function Ψi. This wave function described the behavior of fermion type particles (e.g. electrons) and further predicted the existence of anti particles (e.g positrons) even before they were observed experimentally. The effect of a pre-existing magnetic vector potential {A} and/or a scalar electric potential Φ on the classical Dirac equation is treated by adding additional terms in the free field Dirac PDE’s [1]. COMSOL is used for obtaining a simpler 2-D wave function [Ψ1(x,y,ω),Ψ4(x,y,ω) ] as a solution to the time independent Dirac equation where dependent variables vary as Exp(-iωt) . The probability density ρ(x,y) = |Ψ1|^2 + |Ψ4|^2 of a particle being at a spacial point is treated with the "wave function formulation" which involves solving the electromagnetic Dirac field PDE's for Ψi . USE OF COMSOL MULTIPHYSICS®: The Coefficient-Form PDE "time independent" study is employed. When the wave vector k lies in the xy plane, the 4 component Ψi uncouples into two PDE’s in terms of components for i=1&4. Firstly, PW waveguide examples are solved to test the COMSOL Dirac steady state solving capabilities (in the presence of either a {A} or Φ potential) as compared to alternate FEM or exact solutions, followed by examples with no exact solution available. RESULTS: PW Bar Model: The building block of Dirac theory is the Plane Wave solution, illustrated by the 2-D finite bar (Fig.1a inset), having a normal to xy plane magnetic field vector Bz (with size ~ αB ), which is bounded by an electromagnetic free zone at the start and end of the model. The front end is driven with Ψ1oExp(-iωt) and truncated with PW absorbing B.C. . The Ψ1(x,ω) wave propagation (with gradually increasing spatial frequency) is shown in Fig.1b, illustrating a comparison of the exact (dotted overlay) vs FEM solution. Similar results are solved for Dirac PW’s through Ex electric fields. Two Slit Model: Via two slits, an incident PW enters an infinite domain having a pre-existing in plane Electric field vector Ex, which is bounded by a radial electromagnetic free zone at the start and end of the model. In Fig.1c, waves emerging from the slits interact, forming bands of constructive and destructive interference in a curved fan blade like pattern as opposed to the no Ex field straight blade shaped pattern of the Fig.1d . CONCLUSION: Agreement between the Exact-FEM solution validations were good. The effect of the magnetic or electric field on solutions resulted in Ψi having gradually varying spatial frequencies which in turn effect the spatial shape of Ψ1(x,y),Ψ4(x,y) and hence the probability distribution ρ(x,y) . REFERENCES: [1] Paul Strange, Relativistic Quantum Mechanics, Cambridge University Press Cambridge UK, 1998.