- Bridging the Terahertz Gap
- Modeling the Lithium-Ion Battery
- Protection contre la Corrosion
- Modélisation des batteries
- Modélisation et Simulation dans le développement des piles à combustible
- Modélisation thermique des petits satellites
- Analyse électro-vibroacoustique d'un transducteur à armature équilibrée
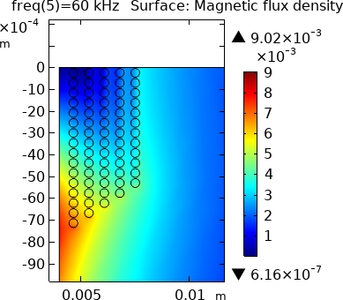
Calculation and Measurement of Winding Loss at High-Frequency Pulsed Currents
Rich literature exists on calculation of copper loss in windings of high-frequency transformers and inductors. Most of the work was carried out in a Dowell approximation ignoring the windings aspect ratio and core presence. Calculation of losses at non-sinusoidal currents is made usually by summing the harmonics losses. This paper makes an example of an inductor with an open ferromagnetic core and a high winding aspect ratio. Simulations were performed on a COMSOL® platform with AC/DC Module, both in frequency and time domains. The latter enable avoiding drudge and inaccuracy of harmonics approximation in the case of periodic signals, and give possibility of calculating losses at arbitrary-shaped currents. The advantages of time-domain approach are distinct when the winding is driven by pulsed current with a low duty cycle D. Methods and simulation results are provided for pulsed sine, rectangular, triangular, and arbitrary shapes at different D’s. First, benchmarking was performed by comparing simulations in the frequency- and time-domains at purely sine waves. It was found that in both simulations the results were very close to each other, and from that, we proceeded to pulsed excitation. It was found that losses at pulsed sine waves were much higher than were those calculated in the frequency domain by summing the losses from up to 11 first harmonics. The rectangular waves generated the highest losses, up to tenfold of those at sine wave. It was also found that the ferromagnetic core increases the loss considerably compared to the case of an air-core inductor, which was confirmed experimentally. The windings resistance was measured with an LCR meter in a wide range of frequencies, up to 1MHz. In addition, adiabatic heating experiments were conducted with the winding driven by DC (for power calibration), sine, and pulsed quasi-sine waves at different frequencies and D’s. The experimental results were found in fair agreement with their theoretical counterparts.