Articles techniques et présentations

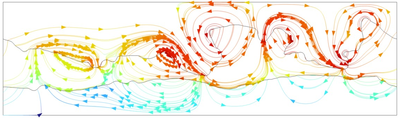
Modern composite membranes for reverse osmosis (RO) and nanofiltration (NF) have an ultrathin polyamide active layer (~100 nm) that performs the ion separation. Current theoretical models explain solute and water fluxes by computing concentration and potential gradients developed in one direction. However, recent experimental studies indicated a relationship between membrane morphology and its permeability and selectivity. We developed a two-dimensional (2-D) model to describe the transport of Na+ and Cl- through RO/NF membranes with irregular polyamide active layer, whose geometry was derived from transmission electron microscopy images. The 2-D model geometry included feed and permeate water domains enclosing an active layer domain (Figure 1). Na+ and Cl- transport in all domains was computed with Tertiary Current Distribution. Ion transport was described by the Electrolyte node in feed and permeate domains and by Ion Exchange Membrane (IEM) in a negatively charged active layer. Velocity field and diffusion coefficients were reduced in the active layer to account for the friction with active layer material. The boundary conditions were: concentrations and electrical insulation at the feed, convection-only and insulation at the permeate, and lateral periodicity. The water/membrane partition was modified from the standard COMSOL® representation of IEM in two aspects. First, the existing Donnan electrostatic barrier was supplemented with a steric hindrance factor, added in the slit boundary Constraints through up() and down() functions (Equation View settings). Second, the continuity conditions for normal ion fluxes and current density had to be modified so that they include also a convective component, not only diffusion and migration (also in Equation View). Finally, the water velocity field supporting the convection was computed with Creeping Flow in the feed and permeate domains, coupled with Darcy's Law in the active layer domain. Pressures values and normal velocities were imposed on the feed and permeate boundaries, lateral boundaries were connected periodically and the interfaces water/active layer were continuous for the flow. The solution strategy consisted of three stationary steps. The water flow was solved first, followed by the mass transport of ions based on the available water velocity field – these two steps being done on a “normal” mesh. In order to improve convergence, the ion transport had to be solved parametrically, by a gradual increase of membrane charge with solution reuse from previous step. Finally, the coupled flow/mass transport solution was improved on a much finer mesh starting from the solution obtained on the normal mesh. Results indicate that the transmembrane pressure and membrane charge are important factors changing the relative dominance of the three ion transport mechanisms. In weakly charged membranes, diffusion is predominant, even at high water fluxes. The electromigration flux can counteract a strong convective flux in highly charged membranes (Figure 2). Unexpectedly, the 2-D model revealed the possible existence of circular ionic currents inside and in the water surrounding the active layer. These currents associated with the Na+ fluxes were typically oriented from feed to permeate in the thinnest regions and cross back through the thickest areas of the active layer (Figure 3).