Articles techniques et présentations

Convection is a method of heat transfer which takes advantage of a fluid’s flow over a solid. At this boundary between the solid and fluid, the convection is quantified by the convective coefficient (h) and the following boundary condition is implemented: [9] k∇T=h∆T where h is the convective coefficient in W/(m^2 k), ∆T is the difference in temperature between the ambient fluid and the solid, k is the thermal conductivity of the solid in W/mk, and ∇T is the temperature gradient. Conventionally, h depends on the physical properties of the fluid and is experimentally determined based on the situation. The purpose of this paper is to predict a value for h as a finned heat sink experiences natural convection. It is important to note the fundamental differences between natural (also referred to as free) convection, and forced convection. In forced convection, the heat transfer is driven by a fluid velocity over the surface of the solid. Often times a fan or pump will create a turbulent flow which correlates to a higher convective heat transfer coefficient and therefore increased heat transfer. For the remainder of this paper, whenever convection is mentioned it is in reference to natural convection. This type of convection is driven by the buoyancy forces created due to temperature differences in the air surrounding a hot surface. The h values for natural convection tend to be h=[2.5,25] W/(m^2 k). The governing equations for modeling natural convection are discussed later in this paper. It is also important to note any assumptions that were taken into account in the following calculations. These assumptions include:

1. Valid boundary layer approximations, no slip, incompressible flow (aside from the varying density, which varies with temperature. As temperature is varying in space, there exists have a density differential, but assume that the total derivative of Dρ/Dt  to be 0).
2. Isothermal fin/base (Since the heat transfer coefficient is very small, it can be assumed assume that temperature variation inside fin does not exist. See eqns 1, 2).
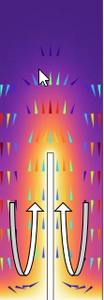
3. Unique inflow/outflow boundary conditions to simulate circulation that occurs as a trademark property of natural convection.

After carrying out the analysis in COMSOL, a reasonable value for h in the heat sink following the geometry above was found to be around 7 W/(m^2 k) which falls within the range of h for natural convection.